Essential Geometry Terms

Geometry shapes our world, influencing everything from architecture to technology. To navigate this world, a solid grasp of geometry terms is crucial. This guide will introduce you to these fundamental terms, explaining them clearly and straightforwardly. We’ll explore essential concepts like points, lines, angles, parallel lines, and shapes, making it easier to understand how geometry applies to everyday life and beyond.

Basic Geometry Terms

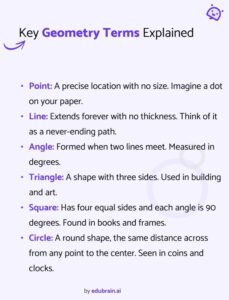
Geometry begins with straightforward concepts. A point marks a specific location, like placing a dot on a piece of paper. A line extends in a very straight line in one direction or path indefinitely without widening.

When two lines intersect, they form an angle, measured in degrees, to describe its sharpness. Lines connecting end-to-end create shapes, with triangles being the most basic, consisting of three sides. If you need help with these concepts, you can use geometry AI for assistance and further explanations.

A square has four equal sides, and every angle between two equal sides is a right angle, like the corners of a square frame. Circles are shapes where all points on the edge are equally distant from the intersection point in the center, much like the shape of most coins.

Lines and Angles Explained

Lines are straight and stretch forever. Some lines, called parallel lines, never meet. They are like railway tracks. Other lines intersect to form a perfect corner on opposite sides. This is a right angle, like the corners on opposite sides of a door. Angles happen where two lines meet.

Here are examples:

• Acute Angle: Less than 90 degrees. Think of the tips of a star.
• Obtuse Angle: More than 90 degrees, but less than 180. It’s like the hands of a clock at 10 o’clock.
• Right Angle: Exactly 90 degrees. They are perfect for making strong corners, like in a square.

Essential Geometry Shapes

Geometry isn’t just about lines and angles; it also includes various shapes foundational to mathematical theory and practical application.

Here are some of the basic geometric shapes:

• Circle: A round shape where all points on the boundary are equidistant from the center. Common examples include wheels and pies.
• Triangle: A three-sided polygon that can vary in side length and angle, making it versatile in both art and engineering.
• Square: A four-sided shape with equal sides and every angle at 90 degrees, like a chessboard square or a window pane.
• Rectangle: Similar to a square, but with varying lengths and widths. Doors and books are often rectangular.
• Polygon: A shape with three or more straight sides. Examples include pentagons, hexagons, and octagons, often seen in tiling patterns.

Complex Geometry Concepts

Advanced geometry introduces concepts that enhance our understanding of space and shapes. A rhombus is a four-sided figure. All its sides are of equal length, but its angles aren’t all right angles. Examples include kites and some types of diamonds. An ellipse looks like a stretched circle. It is the shape of many planetary orbits.

A parallelogram has four sides, too, with opposite ones parallel and equal in length. Its slanted angles make it a common shape in tiles and flooring. A trapezoid has at least one pair of parallel sides. It’s typically used in bridge designs and some furniture. A sphere is a three-dimensional shape, perfectly round from the center at all angles, like a ball or globe.

These words and advanced terms are important for further studies and practical applications in fields like physics and architecture. If you need these words, visual aids or help to solve complex geometry problems, refer to the math image solver. This tool can help you understand and apply these concepts to real-world objects and structures.

Key Theorems and Proofs in Geometry

Geometry involves learning about shapes and rules. Here are some basic rules of solid geometry and formulas:

Pythagorean Theorem

This rule helps in right triangles. It says the longest side squared equals the sum of the squares of the other two sides.

The formula is:

\[
a^2 + b^2 = c^2
\] , where c is the longest side.

Triangle Sum Theorem

This rule says all angles in one direction of a point in a triangle add up to 180 degrees. It helps you find the point with a missing angle.

Area Formulas

These help calculate the space inside shapes. For a rectangle, multiply length by width (Area = length × width).

For a circle, the surface area is \[
\pi r^2
\]
, where r is the radius.

Euler’s Formula

This is for shapes like cubes and pyramids. It connects the number of corners, faces, and edges with the formula: V+F−E=2

These rules are tools for solving problems in building, making, and planning of objects. They make geometry useful in real life.

Practical Uses of Geometry

The subject of geometry is used in many areas of life. Architects and engineers use it to design buildings and bridges. It helps them make structures strong and balanced. Artists and designers also rely on geometry. They use it to create patterns and shapes in their work. Geometry is important in mapping and navigation. It helps make accurate maps and program GPS systems. In healthcare, doctors use geometric algorithms for medical imaging. Scans like MRIs and CTs depend on it to create clear images of the body. If you need help solving geometry issues, try the linear algebra problem solver. It can make tough tasks easier.

Geometry is even used in robotics. Robots use it to plan movements and paths. In gaming, designers use geometry to create realistic worlds and moving objects. These examples show how geometry makes a big impact on everyday life.

Final Thoughts

Geometry is a part of everyday life. We see it in buildings, maps, art, and even robots. Its rules and shapes help us understand and solve problems around us. Learning geometry gives us tools to create, build, and explore.

Whether it’s a plane figure, simple triangle, straight line, curve, or complex design, geometry connects ideas to real-life solutions. It’s not just about math; it’s about understanding the world better. Geometry is everywhere, making life more organized and efficient.